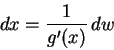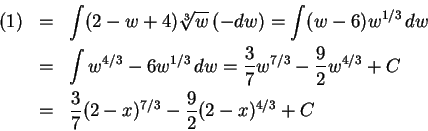積分的變數變換
其實,積分的 變數變換 (change of variables) 技巧,
說穿了還是微分連鎖律的應用,只是當被積分函數
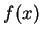 沒那麼容易看成
沒那麼容易看成
則我們令
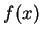 的其中一部份為
的其中一部份為
然後做變數變換
要注意的是 dx 也要變成 dw,方法是
因此我們寫
然後,盡一切努力把被積分函數全部換成 w 變數,
並且祈禱新的積分問題比較簡單。
初學的人不見得很容易找到正確的變換對象。
唯有多練習囉。
以下我們舉一些例子。
這些例子已經不算非常基本的積分技巧,只是放在這裡提供給讀者欣賞淺嚐。
以下的習題,其實比以下所展示的例題,要簡單一些。
- 例一
考慮
令
 則因為
則因為
所以
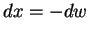 因此
因此
- 例二
- 考慮
令
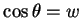 則因為
則因為
所以
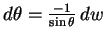 因此
因此
- 例三
- 考慮
令
 則因為
則因為
所以
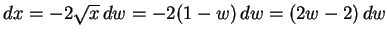 因此
因此
習題
- 請問
- 請問
- 請問
- 請問
- 請問 [HH]
- 請問 [HH]
Created: Dec 11, 1996![]() 沒那麼容易看成
沒那麼容易看成